Investigation #1: Consider again the equation:

Now graph this relation in the xb plane. We get the following graph:

If we take any particular value of b, say b = 5, and overlay this equation on the graph we add a line parallel to the x-axis. If it intersects the curve in the xb plane, the intersection points correspond to the roots of the original equation for that value of b. We have the following graph:

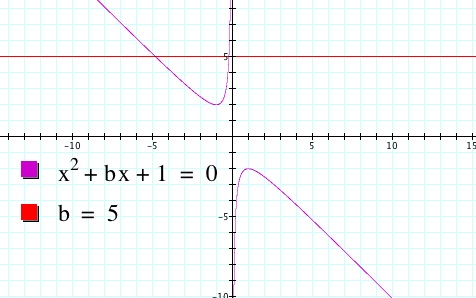
Notice, for each value of b we select, we get a horizontal line. It is clear on a single graph that we get two negative real roots of the original equation when b > 2, one negative real root when b = 2, no real roots for -2 < b < 2, one positive real root when b = -2, and two positive real roots when b < -2. Consider the case when c = -1 rather than +1. Graph other values of c on the same axes.

Investigation:

To begin this investigation, let us consider what the graph would look like if we allow for different values of b. We have an animation that depicts changes in b if we allow b to vary between -5 and 5. It is also helpful to consider the five cases of the number and types of solutions as we vary b,these were provided in the directions for this investigation. See the graph below:

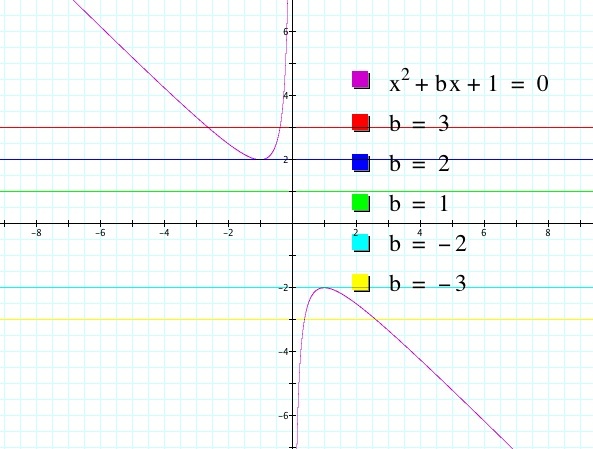
The graph above tells us many things. The example where b = 3 >2, shows us that there are two negative real solutions. The example where b = 2 indiates one negative real solution. When b =1 which allows b to be located within the interval of (-2, 2), we have no real solutions. The horizontal line b = -2, indicates we have one positive real solution. The example where b = -3 < - 2 displays two positive real solutions. Why is this so? Remember standard form for a quadratic equation, where a is the coefficient of the quadratic term, where b is the coefficient of the linear term, and c is the constant term. The equation we are using to explore the xb plane has a = 1 and c = 1. With this information along with our varied b values, we know that we can use these values with the quadratic formula to continue our exploration.

When b = 3 > 2 along with a = 1 and c =1, using the quadratic formula we have the following equation:

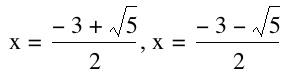
The roots produced by the following equation are approximately x = -0.38 and x = -2.62. Notice, the roots are as predicted from the graph - 2 negative real roots. These values for x are also the x-values associated with the intersection of b = 3 with the original quadratic function in the xb plane. Another interesting observation is a consideration of the value of b and the discriminant. Since b > 2, when a = 1 and c = 1, the value of the discriminant was greater than zero implying two real solutions, and the values for a, b, and c implied that both solutions would be negative.

When b = 2 with a = 1 and c = 1, using the quadratic formula we have the following equation:

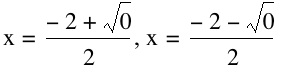
The root produced by the equation above is x = -1. Notice, the root predicted from the graph in the xb plane said that when b = 2 we would have one negative real root, as there was one point of intersection of the line b = 2 with the orginal quadratic equation. The value for x is also the x-value associated with the intersection of the line b = 2 with the orginal quadratic equation in the xb plane. Another interesting observation is a consideration of the value of b and the discriminant. Notice, the discrimination is equal to zero, this results in the exist of one real root. With the discriminant equal to zero, a, b > 0, then we can also say that there is one negative real solution.

When -2 < b =1 < 2 with a = 1 and c = 1, using the quadratic formula we have the following equation:

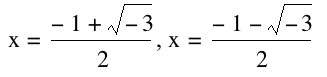
The roots seen here are complex as the discriminant is less than zero. Notice, in the xb plane the line at b = 1 did not have any intersection with the orginal quadratic equation thus implying no real roots. We can also say that when b^2 <4ac, we only have complex roots since the discriminant will be less than zero.

When b = -2 with a = 1 and c = 1, using the quadratic formula we have the following equation:

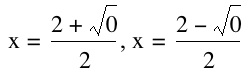
The root produced by this equation above is x = 1. Notice, the root predicted from the graph in the xb plane showed that when b =2 there were one intersections with the orginal quadratic at x = 1. This implied that there was one positive real root with an x-value at x = 1. Similar to the case when b = 2, the value of the discriminant is equal to zero, thus producing only one solution. Notice, it does not matter whether b is positive or negative since within the discriminant the value of b is squared.

Lastly, consider when b =-3 < -2 with a = 1 and c =1, using the quadratic formula we have the following equation:

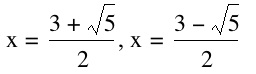
The roots produced by these equations above is x = 0.38 and x = 2.62. This is similar to what we expected when evaluating the intersections seen with b = -3 and the orginial quadratic equation in the xb plane, for the intersections occured when x = 0.38 and x =2.62. We can also observe that one reason that we have two real roots is because b^2 > 4ac, thus implying the discriminant is greater than zero. We have two positive real roots because with the denominator positive and -b > discriminant and -b is greater than zero, we have positive real roots.

Let us consider for a moment what would have if we change the value of c. What if we make c an integer that is less than 0? Look at the following graph of the posibilities.

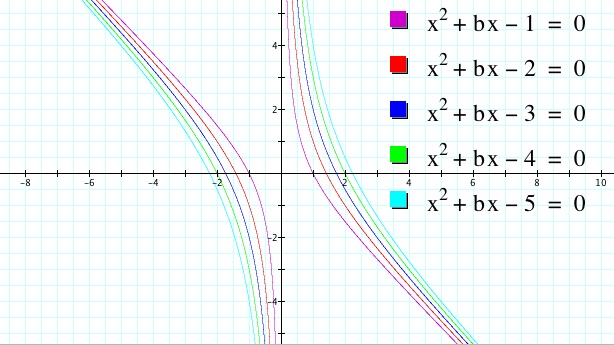
If we allow b =n, how many roots will we have to this equation? Will it always be 2? Will they always be real? Will there always be one positive real root and one negative real root? Consider the quadratic formula and look at this annimation as we vary the value for b.What conjectures can you make?

Return Home